Three different CFGs that each generate the language \(\{abba\}\)
\[\begin{aligned} & ( \{ S, T, V, W\}, \{a,b\}, \{ S \to aT, T \to bV, V \to bW, W \to a\}, S)\\ & \\ & \\ & \\ & ( \{ Q \}, \{a,b\}, \{Q \to abba\}, Q) \\ & \\ & \\ & \\ & ( \{ X,Y \}, \{a,b\}, \{X \to aYa, Y \to bb\}, X) & \\ & \\ \end{aligned}\]
Design a CFG to generate the language \(\{a^n b^n \mid n \geq 0\}\)
Sample derivation:
Design a CFG to generate the language \(\{a^i b^j \mid j \geq i \geq 0\}\)
Sample derivation:
Theorem 2.20: A language is generated by some context-free grammar if and only if it is recognized by some push-down automaton.
Definition: a language is called context-free if it is the language generated by a context-free grammar. The class of all context-free language over a given alphabet \(\Sigma\) is called CFL.
Consequences:
Quick proof that every regular language is context free
To prove closure of the class of context-free languages under a given operation, we can choose either of two modes of proof (via CFGs or PDAs) depending on which is easier
To fully specify a PDA we could give its \(6\)-tuple formal definition or we could give its input alphabet, stack alphabet, and state diagram. An informal description of a PDA is a step-by-step description of how its computations would process input strings; the reader should be able to reconstruct the state diagram or formal definition precisely from such a descripton. The informal description of a PDA can refer to some common modules or subroutines that are computable by PDAs:
PDAs can “test for emptiness of stack” without providing details. How? We can always push a special end-of-stack symbol, \(\$\), at the start, before processing any input, and then use this symbol as a flag.
PDAs can “test for end of input” without providing details. How? We can transform a PDA to one where accepting states are only those reachable when there are no more input symbols.
Over \(\Sigma = \{a,b\}\), let \(L = \{ a^n b^m \mid n \neq m \}\). Goal: Prove \(L\) is context-free.
Suppose \(L_1\) and \(L_2\) are context-free languages over \(\Sigma\). Goal: \(L_1 \cup L_2\) is also context-free.
Approach 1: with PDAs
Let \(M_1 = ( Q_1, \Sigma, \Gamma_1, \delta_1, q_1, F_1)\) and \(M_2 = ( Q_2, \Sigma, \Gamma_2, \delta_2, q_2, F_2)\) be PDAs with \(L(M_1) = L_1\) and \(L(M_2) = L_2\).
Define \(M =\)
Approach 2: with CFGs
Let \(G_1 = (V_1, \Sigma, R_1, S_1)\) and \(G_2 = (V_2, \Sigma, R_2, S_2)\) be CFGs with \(L(G_1) = L_1\) and \(L(G_2) = L_2\).
Define \(G =\)
Suppose \(L_1\) and \(L_2\) are context-free languages over \(\Sigma\). Goal: \(L_1 \circ L_2\) is also context-free.
Approach 1: with PDAs
Let \(M_1 = ( Q_1, \Sigma, \Gamma_1, \delta_1, q_1, F_1)\) and \(M_2 = ( Q_2, \Sigma, \Gamma_2, \delta_2, q_2, F_2)\) be PDAs with \(L(M_1) = L_1\) and \(L(M_2) = L_2\).
Define \(M =\)
Approach 2: with CFGs
Let \(G_1 = (V_1, \Sigma, R_1, S_1)\) and \(G_2 = (V_2, \Sigma, R_2, S_2)\) be CFGs with \(L(G_1) = L_1\) and \(L(G_2) = L_2\).
Define \(G =\)
Summary
Over a fixed alphabet \(\Sigma\), a language \(L\) is regular
iff it is described by some regular expression
iff it is recognized by some DFA
iff it is recognized by some NFA
Over a fixed alphabet \(\Sigma\), a language \(L\) is context-free
iff it is generated by some CFG
iff it is recognized by some PDA
Fact: Every regular language is a context-free language.
Fact: There are context-free languages that are not nonregular.
Fact: There are countably many regular languages.
Fact: There are countably inifnitely many context-free languages.
Consequence: Most languages are not context-free!
Examples of non-context-free languages
\[\begin{aligned} &\{ a^n b^n c^n \mid 0 \leq n , n \in \mathbb{Z}\}\\ &\{ a^i b^j c^k \mid 0 \leq i \leq j \leq k , i \in \mathbb{Z}, j \in \mathbb{Z}, k \in \mathbb{Z}\}\\ &\{ ww \mid w \in \{0,1\}^* \} \end{aligned}\] (Sipser Ex 2.36, Ex 2.37, 2.38)
There is a Pumping Lemma for CFL that can be used to prove a specific language is non-context-free: If \(A\) is a context-free language, there there is a number \(p\) where, if \(s\) is any string in \(A\) of length at least \(p\), then \(s\) may be divided into five pieces \(s = uvxyz\) where (1) for each \(i \geq 0\), \(uv^ixy^iz \in A\), (2) \(|uv|>0\), (3) \(|vxy| \leq p\). We will not go into the details of the proof or application of Pumping Lemma for CFLs this quarter.
A set \(X\) is said to be closed under an operation \(OP\) if, for any elements in \(X\), applying \(OP\) to them gives an element in \(X\).
| True/False | Closure claim |
|---|---|
| True | The set of integers is closed under multiplication. |
| \(\forall x \forall y \left( ~(x \in \mathbb{Z} \wedge y \in \mathbb{Z})\to xy \in \mathbb{Z}~\right)\) | |
| True | For each set \(A\), the power set of \(A\) is closed under intersection. |
| \(\forall A_1 \forall A_2 \left( ~(A_1 \in \mathcal{P}(A) \wedge A_2 \in \mathcal{P}(A) \in \mathbb{Z}) \to A_1 \cap A_2 \in \mathcal{P}(A)~\right)\) | |
| The class of regular languages over \(\Sigma\) is closed under complementation. | |
| The class of regular languages over \(\Sigma\) is closed under union. | |
| The class of regular languages over \(\Sigma\) is closed under intersection. | |
| The class of regular languages over \(\Sigma\) is closed under concatenation. | |
| The class of regular languages over \(\Sigma\) is closed under Kleene star. | |
| The class of context-free languages over \(\Sigma\) is closed under complementation. | |
| The class of context-free languages over \(\Sigma\) is closed under union. | |
| The class of context-free languages over \(\Sigma\) is closed under intersection. | |
| The class of context-free languages over \(\Sigma\) is closed under concatenation. | |
| The class of context-free languages over \(\Sigma\) is closed under Kleene star. | |
Assume \(\Sigma = \{0,1, \#\}\)
| \(\Sigma^*\) | Regular / nonregular and context-free / not context-free |
| \(\{0^i\# 1^j \mid i \geq 0, j \geq 0\}\) | Regular / nonregular and context-free / not context-free |
| \(\{0^i1^j\# 1^j0^i \mid i \geq 0, j \geq 0\}\) | Regular / nonregular and context-free / not context-free |
| \(\{0^i1^j\# 0^i 1^j \mid i \geq 0, j \geq 0\}\) | Regular / nonregular and context-free / not context-free |
Turing machines: unlimited read + write memory, unlimited time (computation can proceed without “consuming” input and can re-read symbols of input)
Division betweeen program (CPU, state diagram) and data
Unbounded memory gives theoretical limit to what modern computation (including PCs, supercomputers, quantum computers) can achieve
State diagram formulation is simple enough to reason about (and diagonalize against) while expressive enough to capture modern computation
For Turing machine \(M= (Q, \Sigma, \Gamma, \delta, q_0, q_{accept}, q_{reject})\) the computation of \(M\) on a string \(w\) over \(\Sigma\) is:
Read/write head starts at leftmost position on tape.
Input string is written on \(|w|\)-many leftmost cells of tape, rest of the tape cells have the blank symbol. Tape alphabet is \(\Gamma\) with \(\textvisiblespace\in \Gamma\) and \(\Sigma \subseteq \Gamma\). The blank symbol \(\textvisiblespace \notin \Sigma\).
Given current state of machine and current symbol being read at the tape head, the machine transitions to next state, writes a symbol to the current position of the tape head (overwriting existing symbol), and moves the tape head L or R (if possible). Formally, transition function is \[\delta: Q\times \Gamma \to Q \times \Gamma \times \{L, R\}\]
Computation ends if and when machine enters either the accept or the reject state. This is called halting. Note: \(q_{accept} \neq q_{reject}\).
The language recognized by the Turing machine \(M\), is \[\{ w \in \Sigma^* \mid \textrm{computation of $M$ on $w$ halts after entering the accept state}\} = \{ w \in \Sigma^* \mid w \textrm{ is accepted by } M\}\]
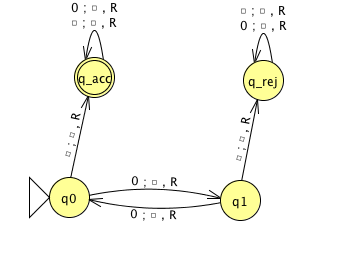
An example Turing machine: \(\Sigma = \phantom{\hspace{1in}}, \Gamma = \phantom{\hspace{1in}}\) \[\delta ( ( q0, 0) ) =\]

Formal definition:
Sample computation:
| \(q0\downarrow\) | ||||||
|---|---|---|---|---|---|---|
| \(0\) | \(0\) | \(0\) | \(\textvisiblespace\) | \(\textvisiblespace\) | \(\textvisiblespace\) | \(\textvisiblespace\) |
The language recognized by this machine is …
Extra practice:
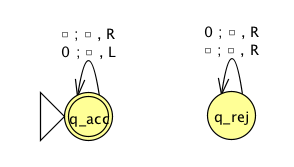
Formal definition:
Sample computation: