nondeterminism

The next model of computation. Idea: allow some memory of unbounded size. How?

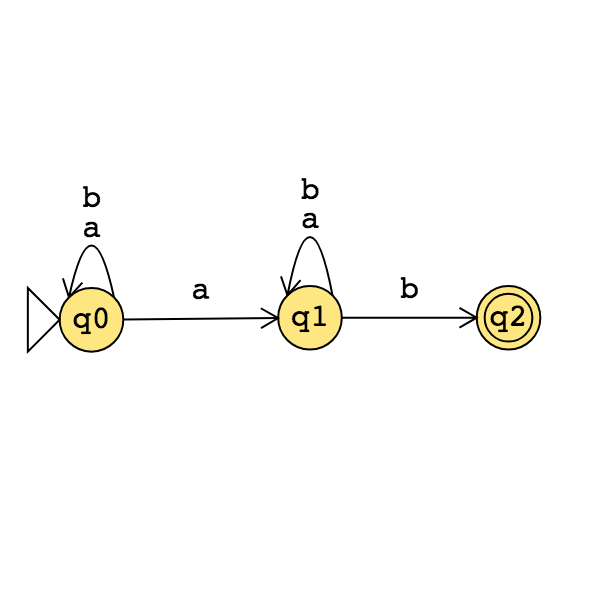
Is there a PDA that recognizes the nonregular language $\{0^n1^n \mid n \geq 0 \}$?

A PDA recognizing the set $\{ \hspace{1.5 in} \}$ can be informally described as:

Week4 wednesday

Definition A pushdown automaton (PDA) is specified by a $6$-tuple $(Q, \Sigma, \Gamma, \delta, q_0, F)$ where $Q$ is the finite set of states, $\Sigma$ is the input alphabet, $\Gamma$ is the stack alphabet, \[\delta: Q \times \Sigma_\varepsilon \times \Gamma_\varepsilon \to \mathcal{P}( Q \times \Gamma_\varepsilon)\] is the transition function, $q_0 \in Q$ is the start state, $F \subseteq Q$ is the set of accept states.

Draw the state diagram and give the formal definition of a PDA with $\Sigma = \Gamma$.

Draw the state diagram and give the formal definition of a PDA with $\Sigma \cap \Gamma = \emptyset$.

Mathematical description of language	State diagram of PDA recognizing language
	$\Gamma = \{ \$, \#\}$




	$\Gamma = \{ \sun, 1\}$







$\{ 0^i 1^j 0^k \mid i,j,k \geq 0 \}$

Week3 monday

Our goals for today are (1) prove similar results about other set operations, (2) prove that NFA and DFA are equally expressive, and therefore (3) define an important class of languages.

Suppose $A_1, A_2$ are languages over an alphabet $\Sigma$. Claim: if there is a NFA $N_1$ such that $L(N_1) = A_1$ and NFA $N_2$ such that $L(N_2) = A_2$, then there is another NFA, let’s call it $N$, such that $L(N) = A_1 \circ A_2$.

Proof idea: Allow computation to move between $N_1$ and $N_2$ “spontaneously" when reach an accepting state of $N_1$, guessing that we’ve reached the point where the two parts of the string in the set-wise concatenation are glued together.

Formal construction: Let $N_1 = (Q_1, \Sigma, \delta_1, q_1, F_1)$ and $N_2 = (Q_2, \Sigma, \delta_2,q_2, F_2)$ and assume $Q_1 \cap Q_2 = \emptyset$. Construct $N = (Q, \Sigma, \delta, q_0, F)$ where

Proof of correctness would prove that $L(N) = A_1 \circ A_2$ by considering an arbitrary string accepted by $N$, tracing an accepting computation of $N$ on it, and using that trace to prove the string can be written as the result of concatenating two strings, the first in $A_1$ and the second in $A_2$; then, taking an arbitrary string in $A_1 \circ A_2$ and proving that it is accepted by $N$. Details left for extra practice.

Suppose $A$ is a language over an alphabet $\Sigma$. Claim: if there is a NFA $N$ such that $L(N) = A$, then there is another NFA, let’s call it $N'$, such that $L(N') = A^*$.

Proof idea: Add a fresh start state, which is an accept state. Add spontaneous moves from each (old) accept state to the old start state.

Formal construction: Let $N = (Q, \Sigma, \delta, q_1, F)$ and assume $q_0 \notin Q$. Construct $N' = (Q', \Sigma, \delta', q_0, F')$ where

Proof of correctness would prove that $L(N') = A^*$ by considering an arbitrary string accepted by $N'$, tracing an accepting computation of $N'$ on it, and using that trace to prove the string can be written as the result of concatenating some number of strings, each of which is in $A$; then, taking an arbitrary string in $A^*$ and proving that it is accepted by $N'$. Details left for extra practice.

Application: A state diagram for a NFA over $\Sigma = \{a,b\}$ that recognizes $L (( a^*b)^* )$:

Suppose $A$ is a language over an alphabet $\Sigma$. Claim: if there is a NFA $N$ such that $L(N) = A$ then there is a DFA $M$ such that $L(M) = A$.

Proof idea: States in $M$ are “macro-states" – collections of states from $N$ – that represent the set of possible states a computation of $N$ might be in.

Formal construction: Let $N = (Q, \Sigma, \delta, q_0, F)$. Define \[M = (~ \mathcal{P}(Q), \Sigma, \delta', q', \{ X \subseteq Q \mid X \cap F \neq \emptyset \}~ )\] where $q' = \{ q \in Q \mid \text{$q = q_0$ or is accessible from $q_0$ by spontaneous moves in $N$} \}$ and \[\delta' (~(X, x)~) = \{ q \in Q \mid q \in \delta( ~(r,x)~) ~\text{for some $r \in X$ or is accessible from such an $r$ by spontaneous moves in $N$} \}\]

Consider the state diagram of an NFA over $\{a,b\}$. Use the “macro-state” construction to find an equivalent DFA.

Consider the state diagram of an NFA over $\{0,1\}$. Use the “macro-state” construction to find an equivalent DFA.

Note: We can often prune the DFAs that result from the “macro-state” constructions to get an equivalent DFA with fewer states (e.g. only the “macro-states" reachable from the start state).

There is a DFA over $\Sigma$ that recognizes $L$	$\exists M ~(M \textrm{ is a DFA and } L(M) = A)$
if and only if
There is a NFA over $\Sigma$ that recognizes $L$	$\exists N ~(N \textrm{ is a NFA and } L(N) = A)$
if and only if
There is a regular expression over $\Sigma$ that describes $L$	$\exists R ~(R \textrm{ is a regular expression and } L(R) = A)$

A language is called regular when any (hence all) of the above three conditions are met.

We already proved that DFAs and NFAs are equally expressive. It remains to prove that regular expressions are too.

Part 1: Suppose $A$ is a language over an alphabet $\Sigma$. If there is a regular expression $R$ such that $L(R) = A$, then there is a NFA, let’s call it $N$, such that $L(N) = A$.

Structural induction: Regular expression is built from basis regular expressions using inductive steps (union, concatenation, Kleene star symbols). Use constructions to mirror these in NFAs.

Application: A state diagram for a NFA over $\{a,b\}$ that recognizes $L(a^* (ab)^*)$:

Part 2: Suppose $A$ is a language over an alphabet $\Sigma$. If there is a DFA $M$ such that $L(M) = A$, then there is a regular expression, let’s call it $R$, such that $L(R) = A$.

Proof idea: Trace all possible paths from start state to accept state. Express labels of these paths as regular expressions, and union them all.

Application: Find a regular expression describing the language recognized by the DFA with state diagram

Week2 friday

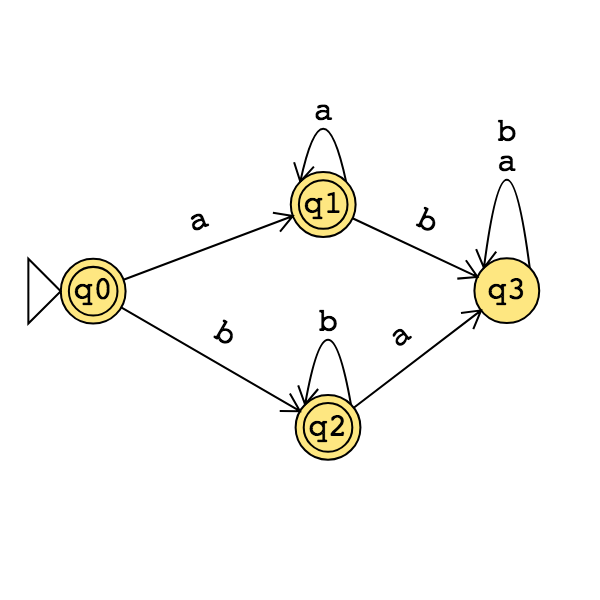
Review: The language recognized by the NFA over $\{a,b\}$ with state diagram

Suppose $A_1, A_2$ are languages over an alphabet $\Sigma$. Claim: if there is a DFA $M_1$ such that $L(M_1) = A_1$ and DFA $M_2$ such that $L(M_2) = A_2$, then there is another DFA, let’s call it $M$, such that $L(M) = A_1 \cup A_2$. Theorem 1.25 in Sipser, page 45

Example: When $A_1 = \{w \mid w~\text{has an $a$ and ends in $b$} \}$ and $A_2 = \{ w \mid w~\text{is of even length} \}$.

Week10 monday

In practice, computers (and Turing machines) don’t have infinite tape, and we can’t afford to wait unboundedly long for an answer. “Decidable" isn’t good enough - we want “Efficiently decidable".

For a given algorithm working on a given input, how long do we need to wait for an answer? How does the running time depend on the input in the worst-case? average-case? We expect to have to spend more time on computations with larger inputs.

Definition (Sipser 7.1): For $M$ a deterministic decider, its running time is the function $f: \mathbb{N} \to \mathbb{N}$ given by \[f(n) = \text{max number of steps $M$ takes before halting, over all inputs of length $n$}\]

Definition (Sipser 7.7): For each function $t(n)$, the time complexity class $TIME(t(n))$, is defined by \[TIME( t(n)) = \{ L \mid \text{$L$ is decidable by a Turing machine with running time in $O(t(n))$} \}\]

Definition (Sipser 7.12) : $P$ is the class of languages that are decidable in polynomial time on a deterministic 1-tape Turing machine \[P = \bigcup_k TIME(n^k)\]

Theorem (Sipser 7.8): Let $t(n)$ be a function with $t(n) \geq n$. Then every $t(n)$ time deterministic multitape Turing machine has an equivalent $O(t^2(n))$ time deterministic 1-tape Turing machine.

Definitions (Sipser 7.1, 7.7, 7.12): For $M$ a deterministic decider, its running time is the function $f: \mathbb{N} \to \mathbb{N}$ given by \[f(n) = \text{max number of steps $M$ takes before halting, over all inputs of length $n$}\] For each function $t(n)$, the time complexity class $TIME(t(n))$, is defined by \[TIME( t(n)) = \{ L \mid \text{$L$ is decidable by a Turing machine with running time in $O(t(n))$} \}\] $P$ is the class of languages that are decidable in polynomial time on a deterministic 1-tape Turing machine \[P = \bigcup_k TIME(n^k)\]

Definition (Sipser 7.9): For $N$ a nodeterministic decider. The running time of $N$ is the function $f: \mathbb{N} \to \mathbb{N}$ given by \[f(n) = \text{max number of steps $N$ takes on any branch before halting, over all inputs of length $n$}\]

Definition (Sipser 7.21): For each function $t(n)$, the nondeterministic time complexity class $NTIME(t(n))$, is defined by \[NTIME( t(n)) = \{ L \mid \text{$L$ is decidable by a nondeterministic Turing machine with running time in $O(t(n))$} \}\]

Brute-force (worst-case exponential time) approach: iterate over all possible solutions, for each one, check if it works.

Week10 wednesday

Can’t use nondeterminism; Can use multiple tapes; Often need to be “more clever” than naïve / brute force approach \[PATH = \{\langle G,s,t\rangle \mid \textrm{$G$ is digraph with $n$ nodes there is path from s to t}\}\] Use breadth first search to show in $P$ \[RELPRIME = \{ \langle x,y\rangle \mid \textrm{$x$ and $y$ are relatively prime integers}\}\] Use Euclidean Algorithm to show in $P$ \[L(G) = \{w \mid \textrm{$w$ is generated by $G$}\}\] (where $G$ is a context-free grammar). Use dynamic programming to show in $P$.

“Verifiable" i.e. NP, Can be decided by a nondeterministic TM in polynomial time, best known deterministic solution may be brute-force, solution can be verified by a deterministic TM in polynomial time.

\[HAMPATH = \{\langle G,s,t \rangle \mid \textrm{$G$ is digraph with $n$ nodes, there is path from $s$ to $t$ that goes through every node exactly once}\}\] \[VERTEX-COVER = \{ \langle G,k\rangle \mid \textrm{$G$ is an undirected graph with $n$ nodes that has a $k$-node vertex cover}\}\] \[CLIQUE = \{ \langle G,k\rangle \mid \textrm{$G$ is an undirected graph with $n$ nodes that has a $k$-clique}\}\] \[SAT =\{ \langle X \rangle \mid \textrm{$X$ is a satisfiable Boolean formula with $n$ variables}\}\]

Problems in $P$	Problems in $NP$
(Membership in any) regular language	Any problem in $P$
(Membership in any) context-free language
$A_{DFA}$	$SAT$
$E_{DFA}$	$CLIQUE$
$EQ_{DFA}$	$VERTEX-COVER$
$PATH$	$HAMPATH$
$RELPRIME$	$\ldots$
$\ldots$

Notice: $NP \subseteq \{ L \mid L \text{ is decidable} \}$ so $A_{TM} \notin NP$

One approach to trying to answer it is to look for hardest problems in $NP$ and then (1) if we can show that there are efficient algorithms for them, then we can get efficient algorithms for all problems in $NP$ so $P = NP$, or (2) these problems might be good candidates for showing that there are problems in $NP$ for which there are no efficient algorithms.

Definition (Sipser 7.29) Language $A$ is polynomial-time mapping reducible to language $B$, written $A \leq_P B$, means there is a polynomial-time computable function $f: \Sigma^* \to \Sigma^*$ such that for every $x \in \Sigma^*$ \[x \in A \qquad \text{iff} \qquad f(x) \in B.\] The function $f$ is called the polynomial time reduction of $A$ to $B$.

Definition (Sipser 7.34; based in Stephen Cook and Leonid Levin’s work in the 1970s): A language $B$ is NP-complete means (1) $B$ is in NP and (2) every language $A$ in $NP$ is polynomial time reducible to $B$.

Theorem (Sipser 7.35): If $B$ is NP-complete and $B \in P$ then $P = NP$.

Week10 friday

3SAT: A literal is a Boolean variable (e.g. $x$) or a negated Boolean variable (e.g. $\bar{x}$). A Boolean formula is a 3cnf-formula if it is a Boolean formula in conjunctive normal form (a conjunction of disjunctive clauses of literals) and each clause has three literals. \[3SAT = \{ \langle \phi \rangle \mid \text{$\phi$ is a satisfiable 3cnf-formula} \}\]

Example string in $3SAT$ \[\langle (x \vee \bar{y} \vee {\bar z}) \wedge (\bar{x} \vee y \vee z) \wedge (x \vee y \vee z) \rangle\]

Example string not in $3SAT$ \[\langle (x \vee y \vee z) \wedge (x \vee y \vee{\bar z}) \wedge (x \vee \bar{y} \vee z) \wedge (x \vee \bar{y} \vee \bar{z}) \wedge (\bar{x} \vee y \vee z) \wedge (\bar{x} \vee y \vee{\bar z}) \wedge (\bar{x} \vee \bar{y} \vee z) \wedge (\bar{x} \vee \bar{y} \vee \bar{z}) \rangle\]

Are there other $NP$-complete problems? To prove that $X$ is $NP$-complete

CLIQUE: A $k$-clique in an undirected graph is a maximally connected subgraph with $k$ nodes. \[CLIQUE = \{ \langle G, k \rangle \mid \text{$G$ is an undirected graph with a $k$-clique} \}\]

Given a Boolean formula in conjunctive normal form with $k$ clauses and three literals per clause, we will map it to a graph so that the graph has a clique if the original formula is satisfiable and the graph does not have a clique if the original formula is not satisfiable.

The graph has $3k$ vertices (one for each literal in each clause) and an edge between all vertices except

Example: $(x \vee \bar{y} \vee {\bar z}) \wedge (\bar{x} \vee y \vee z) \wedge (x \vee y \vee z)$


Model of Computation	Class of Languages


Deterministic finite automata: formal definition, how to design for a given language, how to describe language of a machine? Nondeterministic finite automata: formal definition, how to design for a given language, how to describe language of a machine? Regular expressions: formal definition, how to design for a given language, how to describe language of expression? Also: converting between different models.	Class of regular languages: what are the closure properties of this class? which languages are not in the class? using pumping lemma to prove nonregularity.


Push-down automata: formal definition, how to design for a given language, how to describe language of a machine? Context-free grammars: formal definition, how to design for a given language, how to describe language of a grammar?	Class of context-free languages: what are the closure properties of this class? which languages are not in the class?


Turing machines that always halt in polynomial time	$P$

Nondeterministic Turing machines that always halt in polynomial time	$NP$


Deciders (Turing machines that always halt): formal definition, how to design for a given language, how to describe language of a machine?	Class of decidable languages: what are the closure properties of this class? which languages are not in the class? using diagonalization and mapping reduction to show undecidability


Turing machines formal definition, how to design for a given language, how to describe language of a machine?	Class of recognizable languages: what are the closure properties of this class? which languages are not in the class? using closure and mapping reduction to show unrecognizability

Strategy 3: construct regular expression recognizing the language and prove it works.

Example: $L = \{ w \in \{0,1\}^* \mid \textrm{$w$ has odd number of $1$s or starts with $0$}\}$

Example: Select all and only the options that result in a true statement: “To show a language $A$ is not regular, we can…”

Example: What is the language generated by the CFG with rules \[\begin{aligned} S &\to aSb \mid bY \mid Ya \\ Y &\to bY \mid Ya \mid \varepsilon \end{aligned}\]

Example: Prove that the language $T = \{ \langle M \rangle \mid \textrm{$M$ is a Turing machine and $L(M)$ is infinite}\}$ is undecidable.

Example: Prove that the class of decidable languages is closed under concatenation.

Mathematical description of language	State diagram of PDA recognizing language
	\(\Gamma = \{ \$, \#\}\)




	\(\Gamma = \{ \sun, 1\}\)







\(\{ 0^i 1^j 0^k \mid i,j,k \geq 0 \}\)

There is a DFA over \(\Sigma\) that recognizes \(L\)	\(\exists M ~(M \textrm{ is a DFA and } L(M) = A)\)
if and only if
There is a NFA over \(\Sigma\) that recognizes \(L\)	\(\exists N ~(N \textrm{ is a NFA and } L(N) = A)\)
if and only if
There is a regular expression over \(\Sigma\) that describes \(L\)	\(\exists R ~(R \textrm{ is a regular expression and } L(R) = A)\)